Earlier today I set you three problems, inspired by the 2022 Fields medals. The prizes – which every four years go to up to four mathematicians under 40 – are the most famous award in mathematics.
Maryna Viazovska, from Ukraine, won for her groundbreaking work on how to pack spheres in 24 dimensions. The first puzzle was about how to pack beers in three dimensions.
1. A crate problem
Is it possible to put more than 40 cans of beer of diameter 1 unit and height 2.6 units in a crate that has dimensions 5 x 8 x 2.6?
Solution Yes, it is possible. You can get 41 in by packing in hexagonal fashion
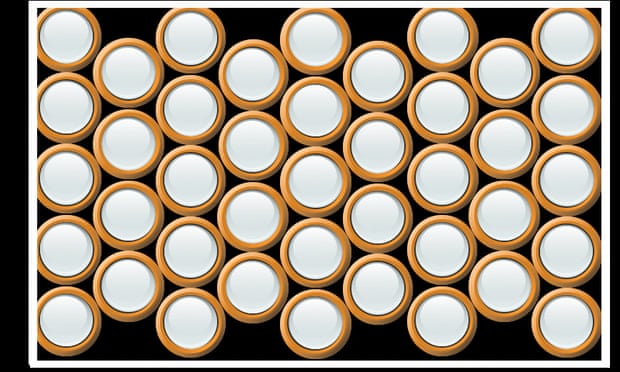
If you don’t have enough cans at home to prove it, Pythagoras can prove it for you. The Pythagorean Theorem states that for right-angled triangles, the square of the hypotenuse equals the sums of the squares on the other two sides. Thus in the triangle below, 12 = x2 + (0.5)2, or x = √(0.75) = 0.87 to two decimal places.
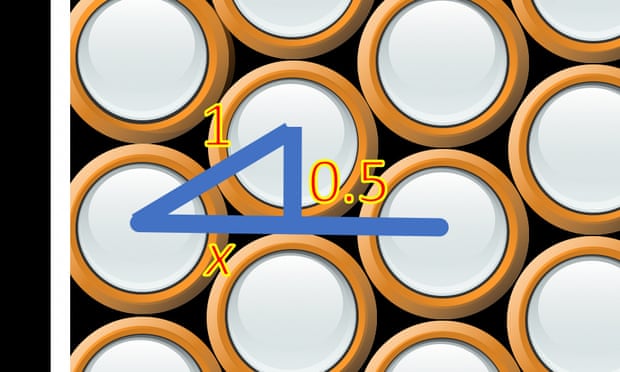
When nine vertical rows (of 41 cans) are stacked, the horizontal distance is 0.5 + 8x + 0.5 = 1 + (8 x 0.87) = 1 + 6.93 = 7.93. This number is less than 8, so we know the cans will fit.
The second puzzle, about the prime number 13, was inspired by Briton James Maynard’s Fields Medal for his many prime results about prime numbers.
2. Chairs, mate.
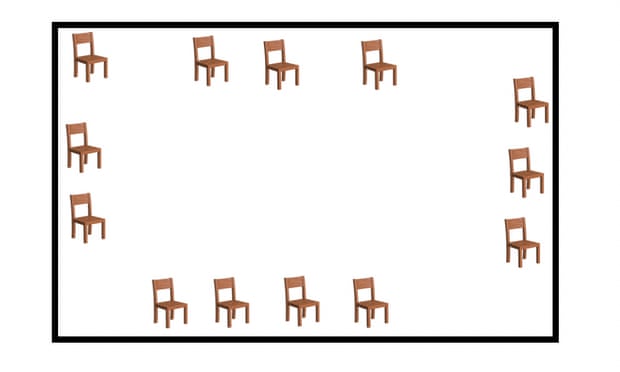
Place 13 chairs along the walls of a rectangular room such that each wall has the same number of chairs as the wall it faces.
Solution
The third puzzle was a tribute to June Huh, whose Fields medal was awarded for results linking graph theory, combinatorics, algebra and many other abstract concepts. A graph in this context means a network of discrete points connected to each other, which is one way you might think of a chessboard, which is discrete squares connected to each other.
3. Chess neighbours
Imagine a 9×9 chessboard. (Like a Sudoku grid, but with alternating black and white cells). Each square has a different person standing on it. Is it possible for all 81 people to step onto a neighbouring square, so that each square again has a different person on it?
Solution No.
If everyone is on a square, this means that 40 people are on one colour of square, and 41 are on the other colour of square. When everyone moves to a neighbouring square, all people on black go to white, and vice versa. But this move is impossible since the number of black squares is not equal to the number of white squares.
I hope you enjoyed today’s puzzles. I’ll be back in two weeks.
PS Don’t forget to buy the Guardian on Saturday (July 16). I have edited a 16-page Summer puzzle supplement that will be free with the physical paper. The supplement includes puzzles from around the world including hand-crafted sudoku by our pals at Cracking the Cryptic, brand new Japanese logic grid puzzles, a selection of teasers from the Grabarchuk family, many different types of word puzzles and several crosswords including a guide of how to solve cryptics. Don’t miss it!
Sources: 1. Trần Phương, 2&3 Half a Century of Pythagoras Magazine.
I set a puzzle here every two weeks on a Monday. I’m always on the look-out for great puzzles. If you would like to suggest one, email me.
I’m the author of several books of puzzles, and also the children’s book series Football School. The latest instalment, The Greatest Ever Quiz Book, is just out.
I give school talks about maths and puzzles (online and in person). If your school is interested please get in touch.

